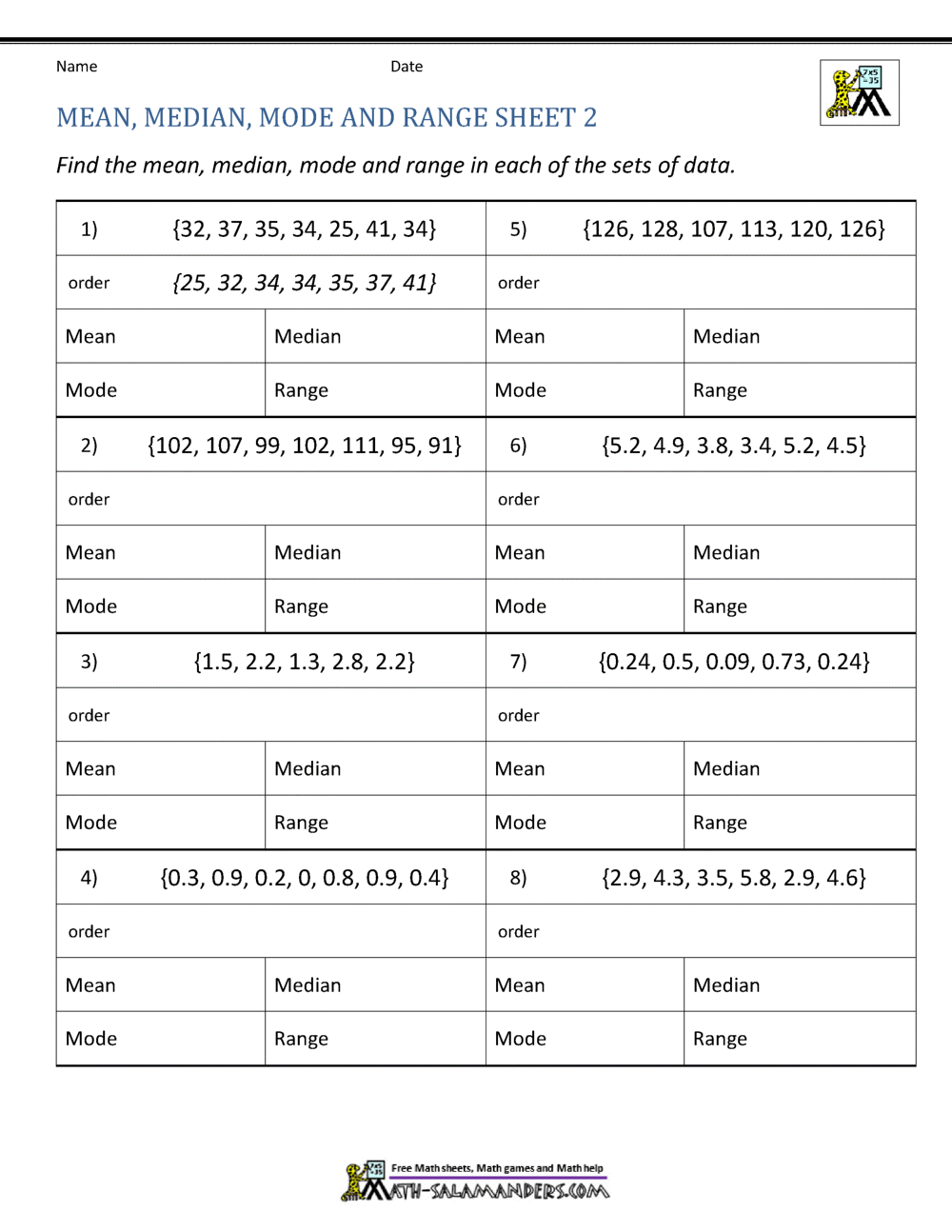
How to Find Mode, Median, Mean and Range: 9 Steps with Pictures

How to Find Mode, Median, Mean and Range: 9 Steps with Pictures

Perfect for homework, in-class activities, or math revision. The decision between mean and median only really matters if the data are skewed. If the data are symmetric, then the mean and median are going to be approximately equal, and the distinction between them is irrelevant. The median is a better measure of the «center» than the mean because 49 of the values are 30,000 and one is 5,000,000.

• The data set is bimodal because the scores 430 and 480 each occur twice.
• If the data set consists of more than one mode then it is known as “multi-modal”(can be bimodal or trimodal).
• Mean, median, and mode are measures of central tendency and are three different ways of expressing averages of a set of data.
• The mean, the median and the mode are three different measures of average which we can use.
• Calculated by adding up all values of a dataset and dividing them by the total number of values in dataset.

Reader Success Stories

The numerical average can mislead by suggesting that the average (in this case, we mean «typical») person earns a few tens of thousands per year. This is an interesting example because the elements in the set now contain zeroes, a positive, and negative numbers. However, the methods that are used to solve for the mean, median, mode, and range do not change. To solve for the median, let’s arrange the list in increasing order and then pick the center value.

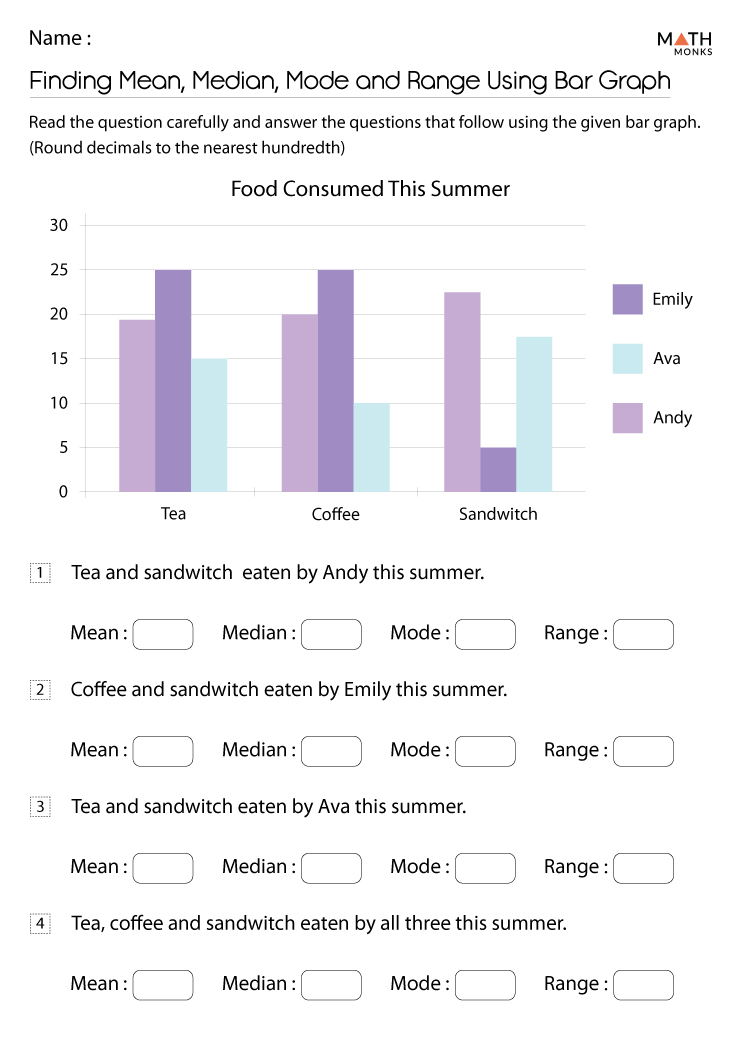
Example 8.18

Clearly, the middle value is 6, so you can conclude that the median of the data set is equal to 6. To determine the median of numbers in the data set, simply find the middle value. To determine the mean of the data set, divide the total sum by the total amount of numbers. Furthermore, the range of a set of data what is range mode median and mean is the difference between the highest and lowest values. In Example 8.3, we created a frequency distribution of the number of siblings of conflict resolution class attendees. Mean, Median, and Mode of any given data set is calculated using the suitable formulas which are discussed above in the articles.

Comparing two sets of data

The median is generally a better measure of the center when there are extreme values or outliers because it is not affected by the precise numerical values of the outliers. Mean, Median, and Mode are measures of the central tendency. These values are used to define the various parameters of the given data set. Mean, median, and mode are measures of central tendency and are three different ways of expressing averages of a set of data. Mean, median, and mode are measures of central tendency used in statistics to summarize a set of data. If you’re trying to identify patterns or trends in a data set, you can use the three measures of central tendency–mean, median, and mode.

Measures of Central Tendency

The location of the median and the value of the median are not the same. The upper case letter \(M\) is often used to represent the median. The next example illustrates the location of the median and the value of the median.

Divide the sum of these values by the total number of data values in the set. The «center» of a data set is also a way of describing location. The two most widely used measures of the «center» of the data are the mean (average) and the median. To calculate the mean weight of 50 people, add the 50 weights together and divide by 50. To find the median weight of the 50 people, order the data and find the number that splits the data into two equal parts.

So, they are the modes (and we can conclude that this set of data is bimodal). The letter “M” is commonly used to represent the median of a dataset, whether it’s for a population or a sample. This notation simplifies the representation of statistical concepts and calculations, making it easier to understand and apply in various contexts. Therefore, in Indian statistical practice, “M” is widely accepted and understood as the symbol for the median. The three measures of central tendency provide different ways of summarizing and describing a set of data. In other words, they can help you to gain a more complete understanding of the data’s distribution.

Statistical software will easily calculate the mean, the median, and the mode. Some graphing calculators can also make these calculations. In the real world, people make these calculations using software.

The mode is the only average that can have no value, one value or more than one value. If there are two middle numbers, the median is the mean of those two numbers. If you had forgotten to divide by 5, then the answer would be 265, which is incorrect. A median value would be better to use if the supermarket didn’t want figures to be affected by unusually higher sales in December, for example.

But there is no «middle» number, because there are an even number of numbers. By quick inspection, the values in this set contain numbers that have different decimal places. Hopefully, you start by wondering how many decimal places should we round off the final answer.

Again, it wouldn’t hurt if you ask advice from your teacher on how many decimals to round off as this part of the solution may be open to different interpretations. It is apparent that no value is repeated more often than the other. It just happens that the two center values are the same, therefore the average of two equal numbers will equal the same number.

Автор публикации

не в сети 3 дня

sbuneass

0

Комментарии: 0Публикации: 1196Регистрация: 21-09-2018